Physics, Algebra, Geometry, Calculus
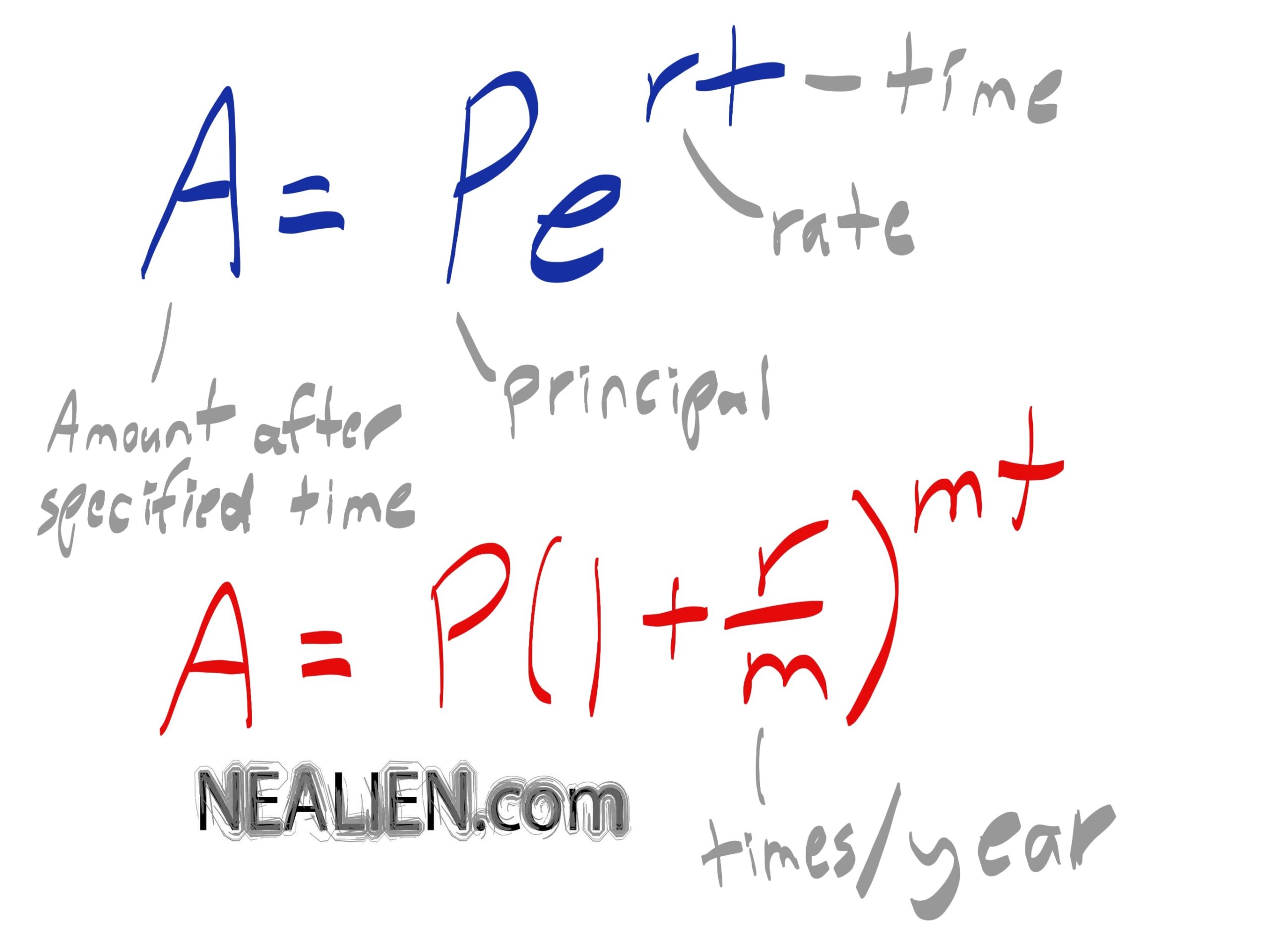
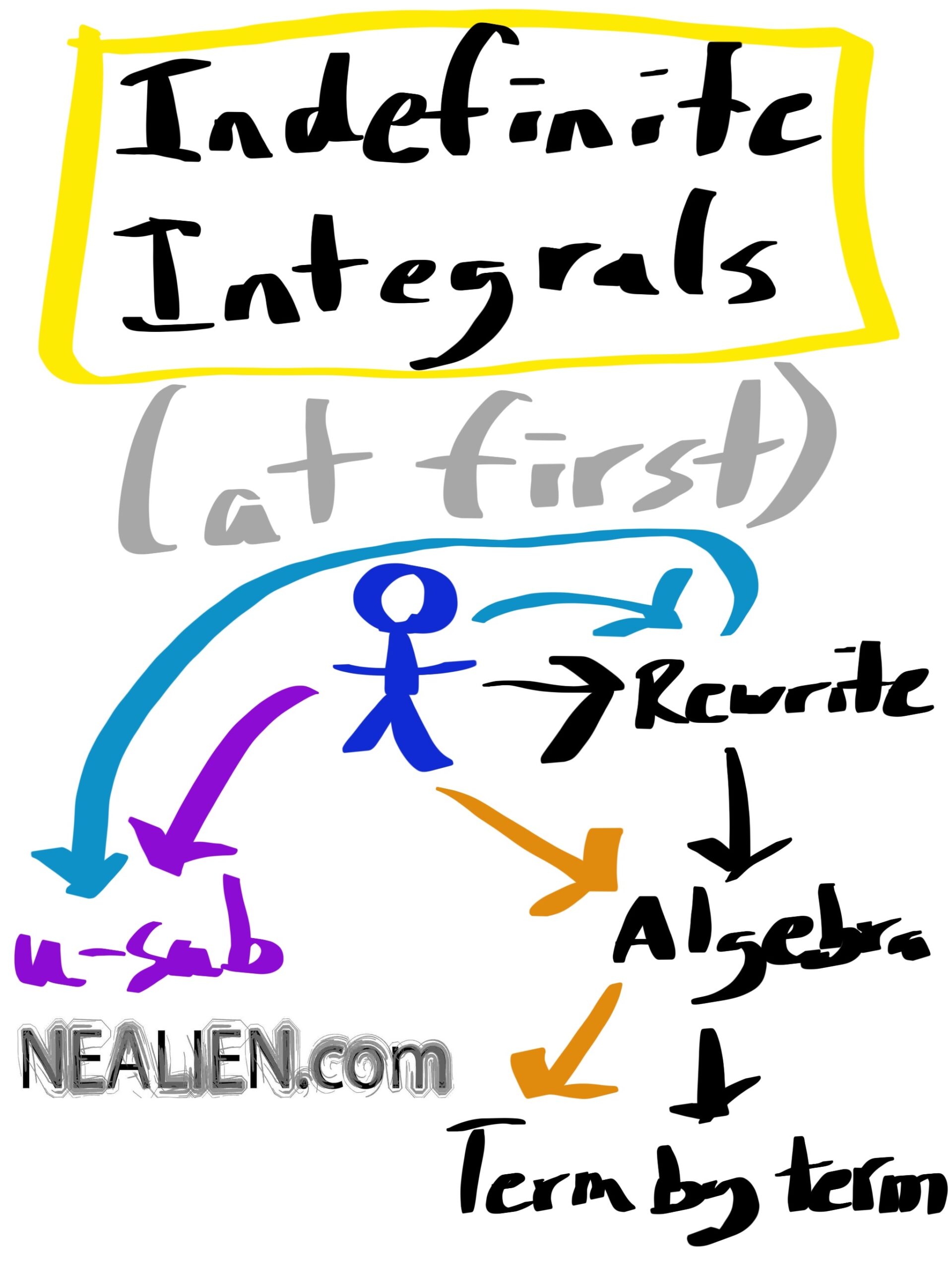
We spent some time looking at linear equations in business situations. Profit, cost, revenue, and demand. They work like similar problems, just with business concepts incorporated. They tend to use …
Continue Reading about Tutoring Business Calculus, Linear Equations in Business Situations →
We mostly went over review problems that had to do with optimization, much like the earlier test. One problem had three variables, therefore it had one primary equation and two secondary equations. …
Continue Reading about Tutoring RLS Calculus, Reviewing Optimization →
Limits mean going towards something. Sometimes you approach a different point from the left and right. Most of the limit problems we saw have the limit of f(x) as x goes to some number, often times …
Continue Reading about Tutoring Business Calculus, Looking more at limits and logs →
Spent a bit of time on problems with the definition of the derivative. It's like getting a slope with the distance between two points being h, and then h goes to zero. At a min or max, the derivative …
Continue Reading about Tutoring Business Calculus, Two types of interest rate equations →
We mostly went over problems with limit. For the types of functions we usually see, if you approach a spot from the left and from the right, the two approaches end up at the same place. When …
Continue Reading about Tutoring Calculus, Looking at limits →
We reviewed for a test today. It focuses on anti-derivatives (indefinite integrals). There are a few options for approaching the problems. With u-substitution, you should be able to see fairly soon …
Continue Reading about Tutoring Calculus – Options for Basic Integrals →
