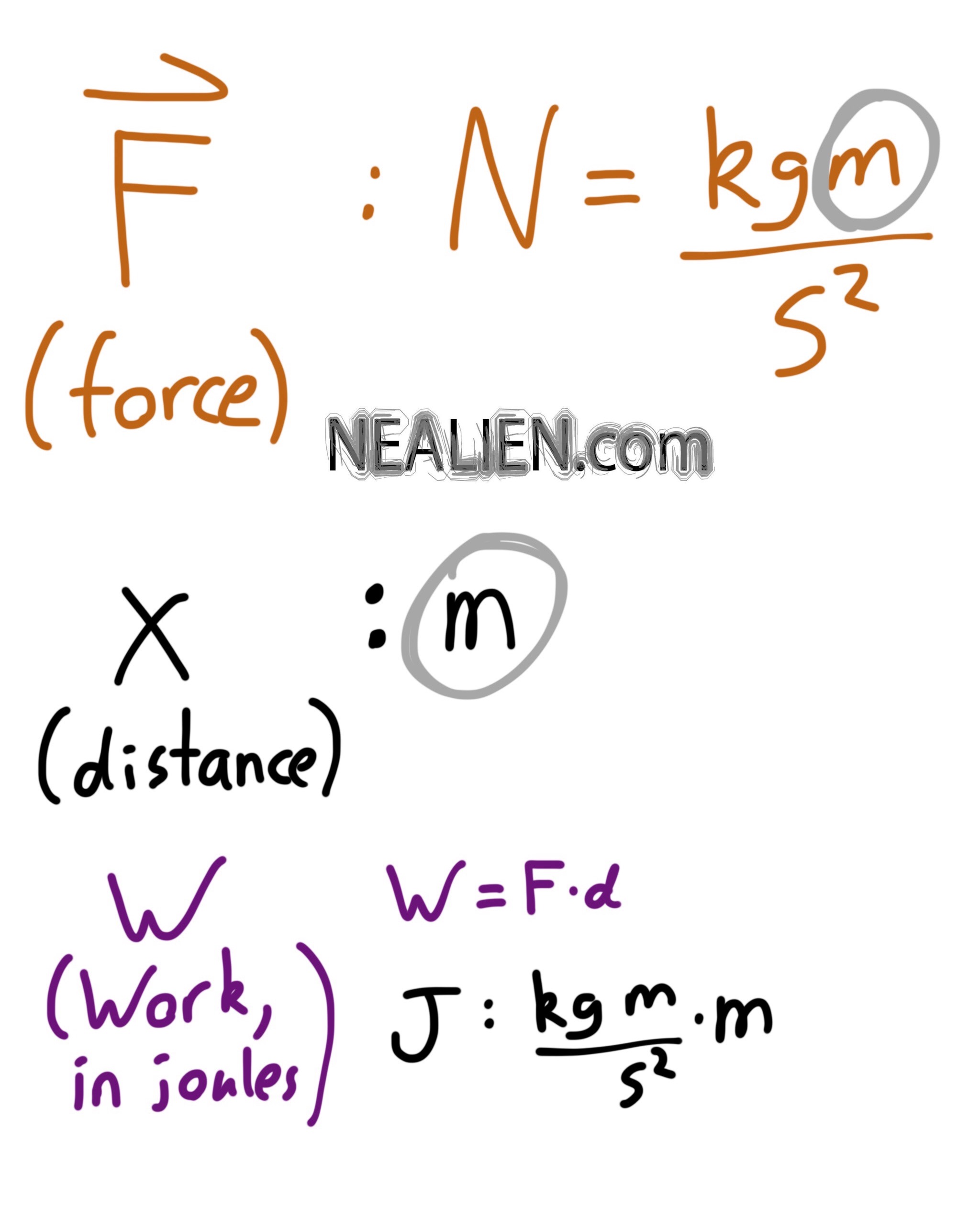
About a week ago I was preparing with a student for a quiz in the Honors Physics class at Carmel High School. The class of Meeps and Moops. We went over the kinematic equations, what …
Continue Reading about Studying for Carmel High School Honors Physics Makeup Quiz →