Physics, Algebra, Geometry, Calculus
Get a quote for help with your classes
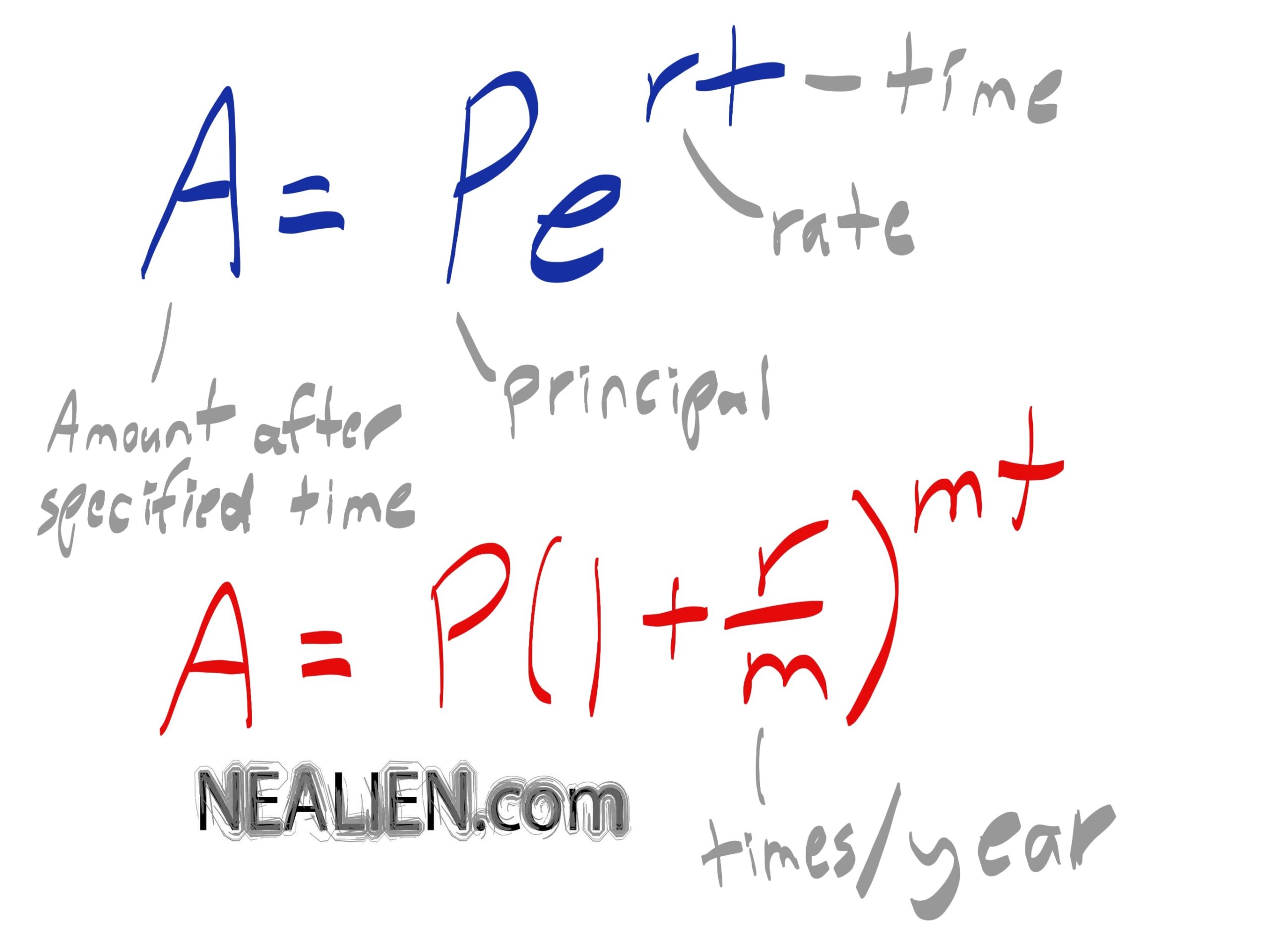
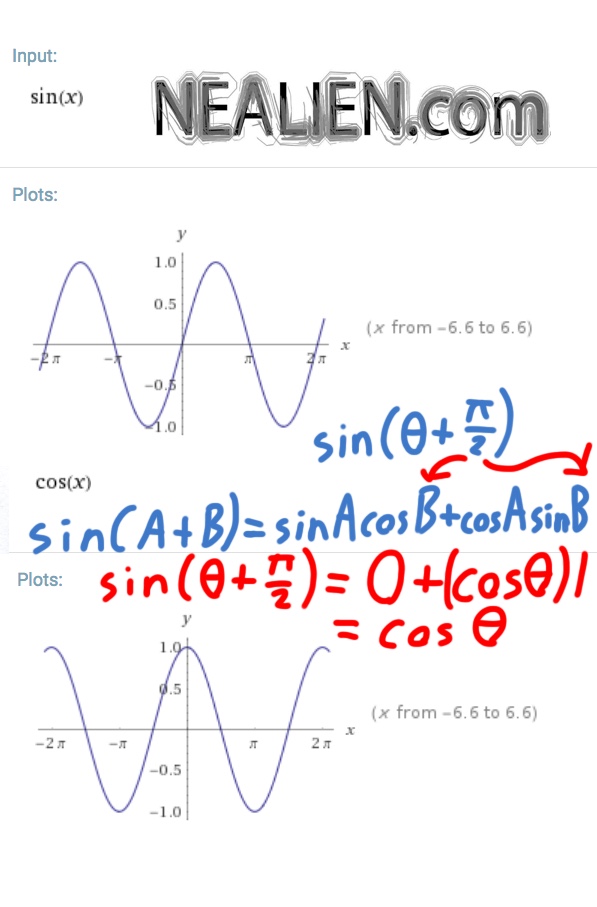
Spent a bit of time on problems with the definition of the derivative. It's like getting a slope with the distance between two points being h, and then h goes to zero. At a min or max, the derivative …
Continue Reading about Tutoring Business Calculus, Two types of interest rate equations →
We reviewed for the upcoming test. Spent some time on basic problems with derivatives. Spent a bit of time on word problems with optimization. There, for a few problems it involved 'showing' that an …
We mostly went over problems with limit. For the types of functions we usually see, if you approach a spot from the left and from the right, the two approaches end up at the same place. When …
Continue Reading about Tutoring Calculus, Looking at limits →
We started by looking at how to combine 'like terms'. Numbers can be added and subtracted, they are 'like'. Also numbers of x or y or z. x^2 and y^2, etc are also like. Looked at factoring …
Continue Reading about Tutoring Prealgebra- combining like terms, dividing by fractions →
If you look at the graphs, you can see that they are different by a phase angle of pi/2. You can also use the equation for sin(A+B) …
Continue Reading about “Why is it that sin(θ+π/2)=cos(θ) for all angles θ?” →
We went through the midterm test. A few concepts were missing in his approach, like absolute value and a mistake with adding negative numbers. Spent a bit of time on changing fractions by multiplying …
Continue Reading about Tutoring Precalculus, Using Reciprocals →
4.9
