Physics, Algebra, Geometry, Calculus
Get a quote for help with your classes
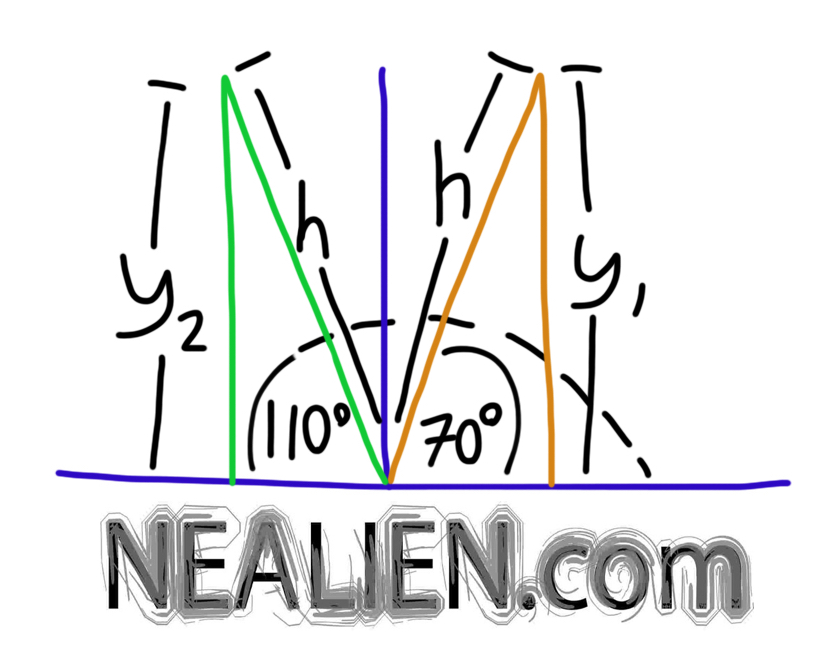
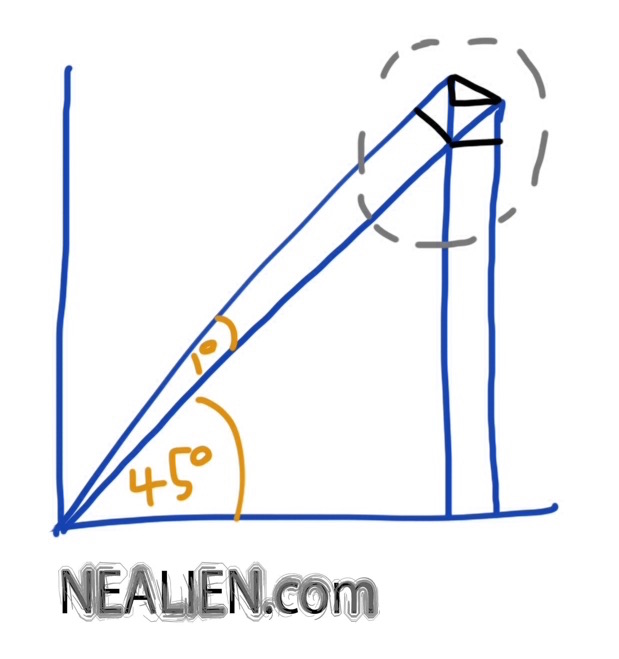
From Quora, Here's a diagram. Each is 20°away from 90°. If you think in terms of the ratios of the sides of the triangles for the angles, SOHCAHTOA, then the opposite sides are the same and the …
Continue Reading about “Why is sin(70°) the same as sin(110°)? →
From Quora, The first book in college (as a physics major) I used for Quantum Mechanics was the book by Griffiths. Introduction to Quantum Mechanics (2nd Edition) David J. Griffiths: 9780131118928: …
Some notes from tutoring today, His class is studying sound waves right now. We looked at questions related to a lab. One question had to do with the accuracy of finding the speed of sound using …
Saw this question on Quora and thought it was interesting. The sun is hot enough to have nuclear fusion. But let's pretend that it was just a fire like we would have burning wood on Earth and …
Like Terry Moore said, it actually is pretty easy to work with in the original form, √96. Sometimes it is better to 'simplify' by getting a coefficient multiplied by a smaller number within the …
Continue Reading about “What is the square root of 96 in radical form?” →
Came up with a bit of a strange method for an approximation of tan(46 degrees) I would first note that 46 degrees is close to 45 degrees. The tan(45 degrees) = 1 since it's a 45 degree triangle and …
Continue Reading about “How will you evaluate the approximate value of tan 46 degrees?” →
4.9
